Solar System
Chung van Gog, 03 October 2022

I love our solar system. And obviously I’m partial to my cuboidal prefixes. So let’s consider some cool solar system facts. :)
Masses
nUUKg
- Sol: 2.0 UUKg

nUNKg
- J: 1.9 UNKg
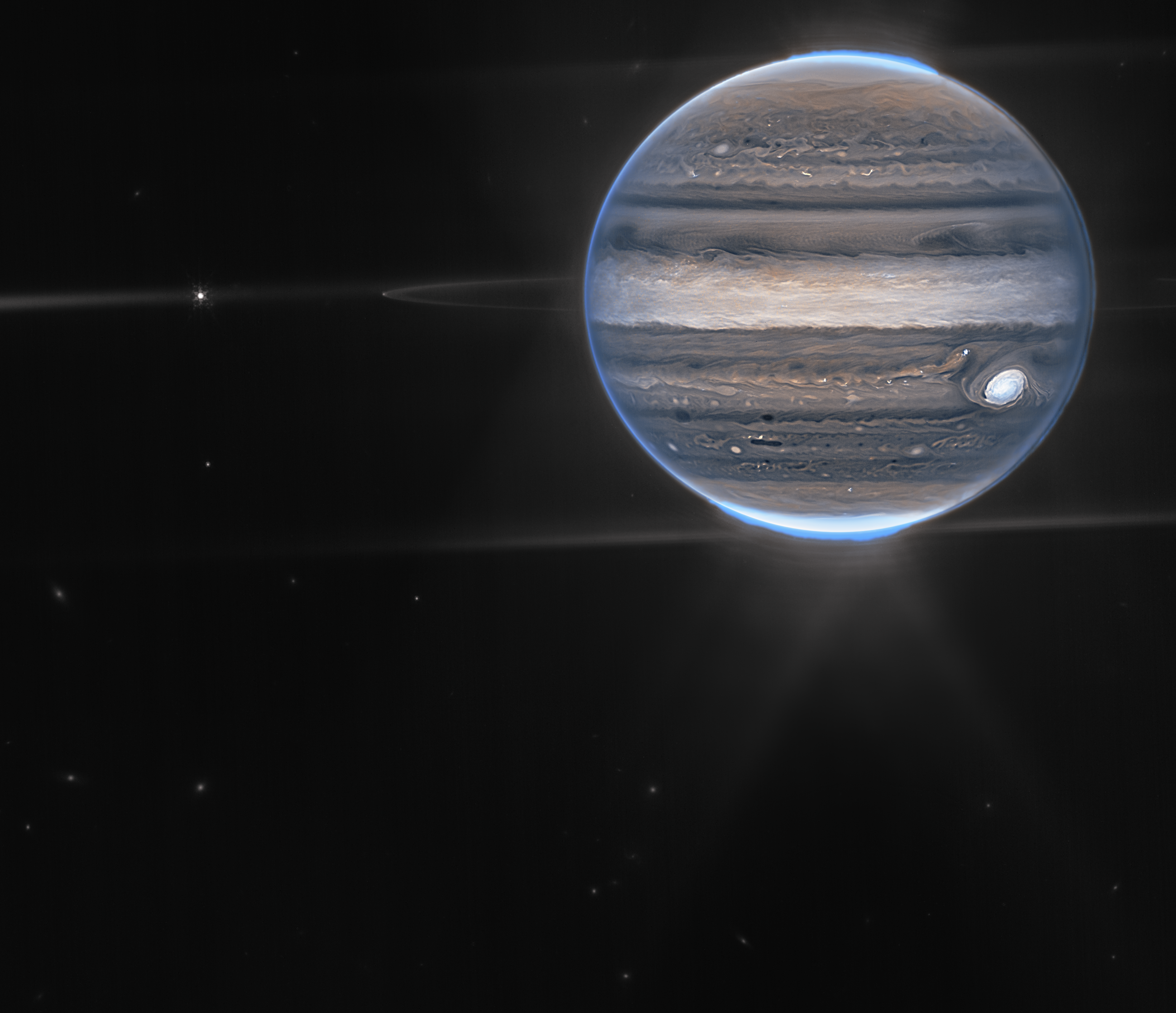
hEKg

daEKg
- U: 87 EKg
- Planet 9 (hypothetical): 30-51 EKg
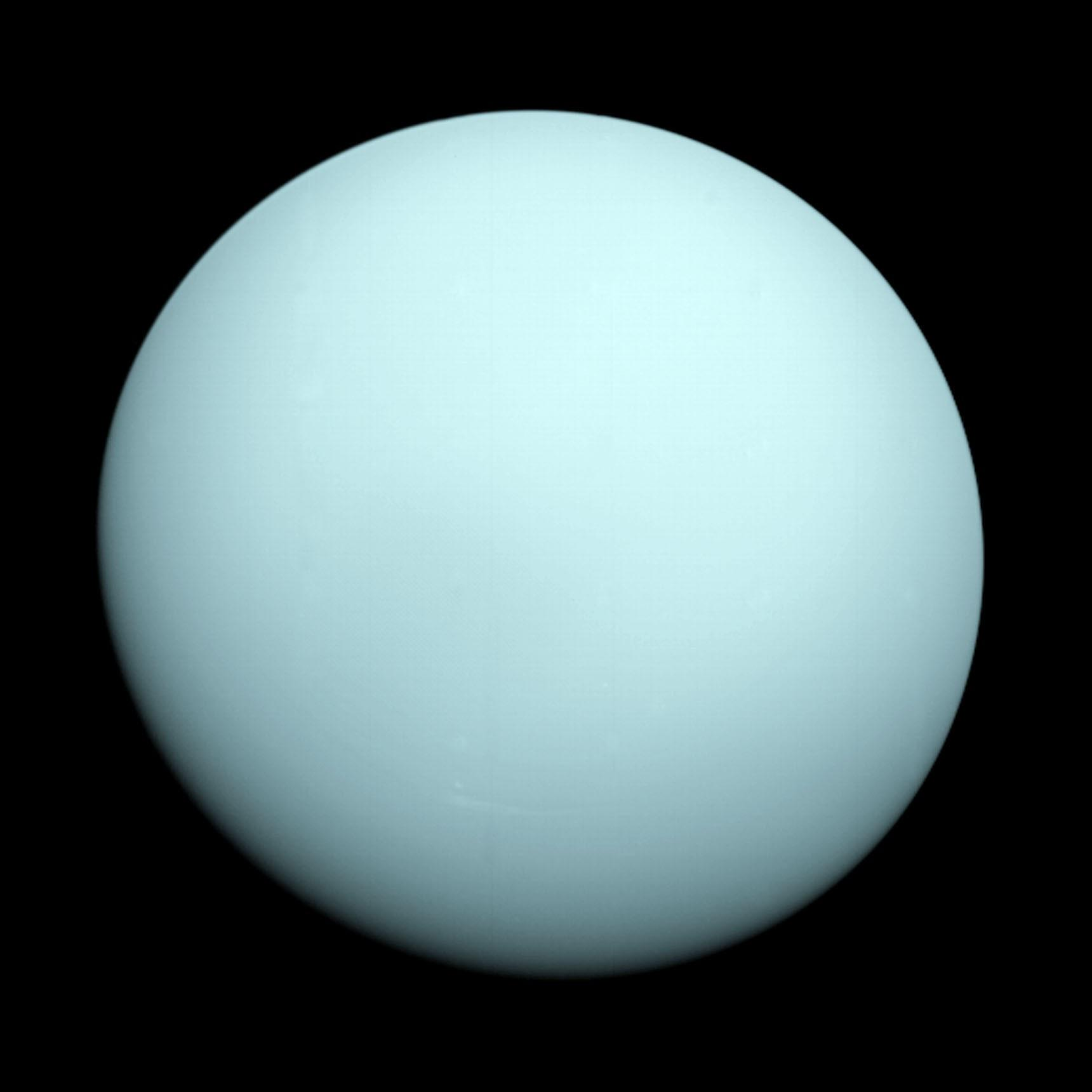
nEKg

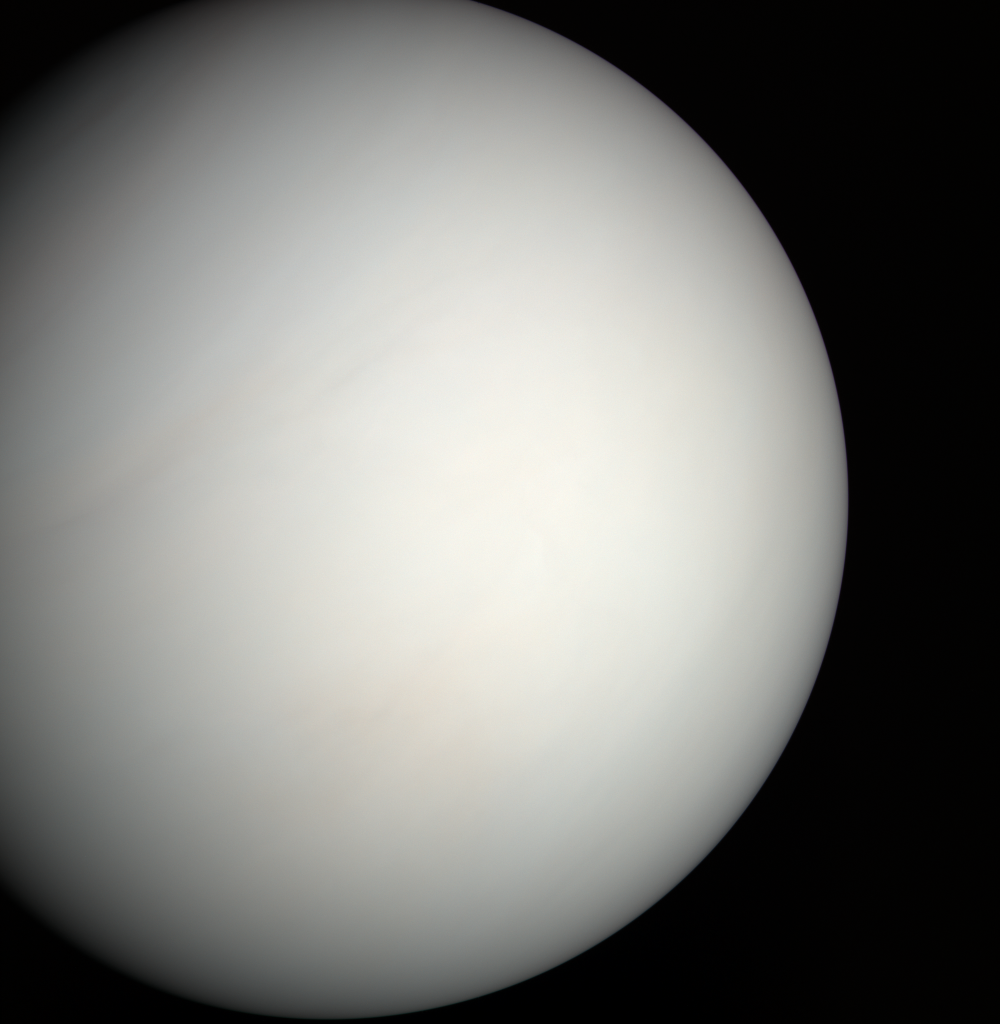
hOKg


daOKg
- J Io: 89 OKg
- E Moon: 73 OKg
- J Europa: 48 OKg
- N Triton: 21 OKg
- Eris: 17 OKg
- Pluto: 13 OKg

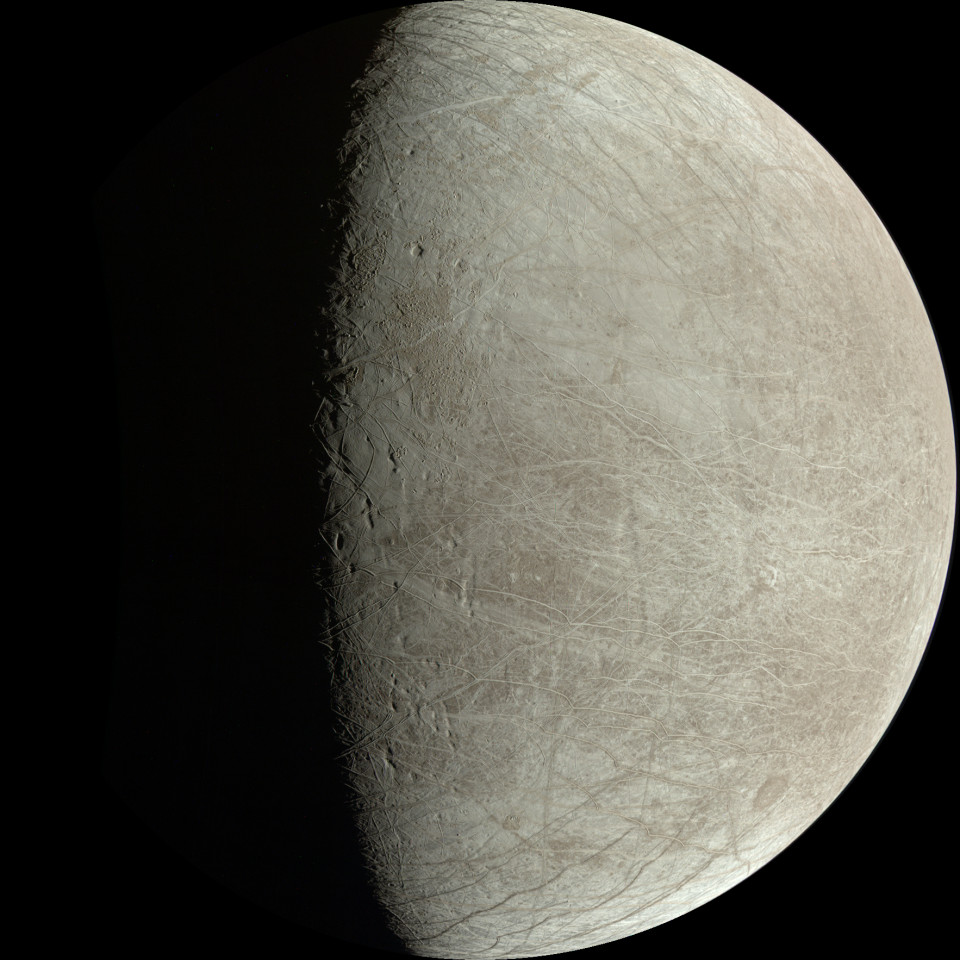
nOKg
- Haumea: 4.0 OKg
- U Titania: 3.4 OKg
- Makemake: 3.1 OKg
- U Oberon: 3.1 OKg
- S Rhea: 2.3 OKg
- S Iapetus: 1.8 OKg
- Gonggong: 1.8 OKg
- Pluto Charon: 1.6 OKg
- Quaoar: 1.4 OKg
- U Umbriel: 1.3 OKg
- U Ariel: 1.3 OKg
- S Dione: 1.1 OKg

Just as an aside, I like thinking of the above as “XKg planets”, with X ≤ UN. So we have one UNKg planet (Jupiter), five EKg planets, and 23+ OKg planets. Usually these are subdivided into planets, dwarf planets, and moons. But why not think in terms of family? Triton, Rhea, Iapetus, and Dione are all OKg planets part of the Neptunian family (Neptune itself being an EKg planet).
Semi-major axes
daTKm
- Me: 58 TKm
hTKm
- V: 108 TKm
- E: 150 TKm
- Ma: 228 TKm
- J: 778 TKm
nQKm
- S: 1.4 QKm
- U: 2.9 QKm
- N: 4.5 QKm
- Pluto: 5.9 QKm
- Haumea: 6.5 QKm
- Quaoar: 6.5 QKm
- Makemake: 6.8 QKm
daQKm
- Eris: 10 QKm
- Gonggong: 10 QKm
- Planet 9 (hypothetical): ~67 QKm
daPKm
- Proxima Centauri: 40 PKm

Orbital periods
These can be calculated with Kepler’s third law:
$T = \tau \sqrt{\frac{a^3}{\mu}}$
Here $T$ is the orbital period in seconds, $\tau = 2\pi$ as usual, $a$ is the semi-major axis, and $\mu = GM_S$ is the gravitational constant times the mass of the Sun. For the solar system, we have $T \approx 5.45408$ $a^{3/2}$ dtms.
So for Earth, we get $\text{(}149.598\text{ TKM)}^{3/2} = 57.8614\text{ PKM}^{3/2}$ and thus $T \approx 365.26$ days, which is pretty close (even if imperfect due to rounding).
